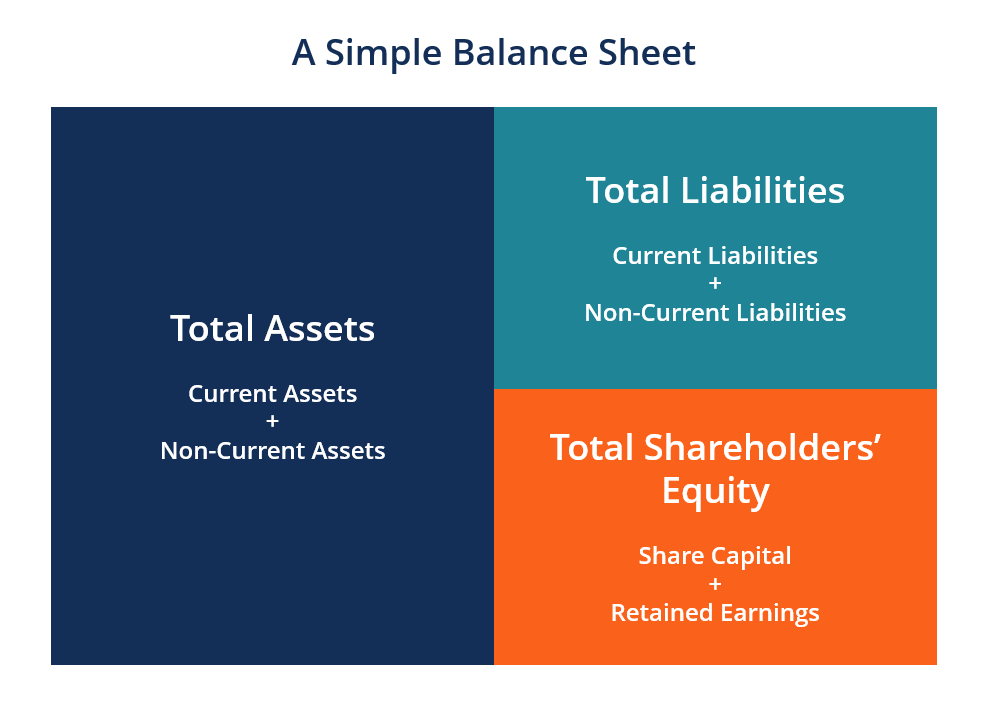
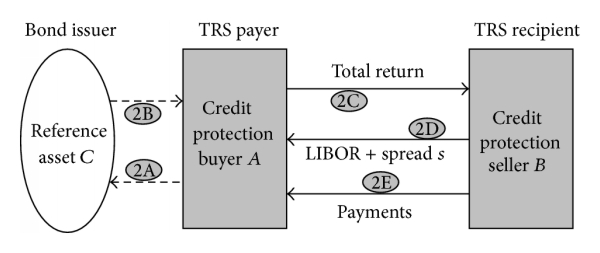
Contents:

This tops the list of all maths properties due to its multiple applications. The distributive property of multiplication can be used when you multiply a number by a sum. For example, suppose you want to multiply 3 by the sum of \(\ 10+2\).
Application and characterization of a novel PVDF-HFP/PVP polymer … – Nature.com
Application and characterization of a novel PVDF-HFP/PVP polymer ….
Posted: Wed, 01 Mar 2023 08:00:00 GMT [source]
In the previous post I talked about using parentheses or brackets to specify this order . This means that the common difference of this arithmetic progression is 5 and thus it is an increasing arithmetic progression. If you see that a sequence has terms that are alternatively positive and negative, then it must be clear from the given sequence that it can never be an arithmetic progression. After subtracting, look at the expression formed. If this expression is a constant number or anything independent of the term n, the sequence is an arithmetic progression.
The https://1investing.in/ answer is \(\ y \cdot 52\). Identify and use the associative properties for addition and multiplication. Overall, arithmetic and it’s basic operations are fundamental in the study of mathematics. The commutative property deals with the order that an addition or multiplication problem is given in. Or we can say for a real number, it reverses its sign from positive number to negative and negative number to positive. The division is the operation that computes the quotient of two numbers.
In words, the logarithm of the product of two numbers is the sum of the logarithms of those numbers . How about multiplication over division? Or any of the other possibilities?
Modular Addition:
And if it arithmetic properties both on the left and on the right, we simply say that distributes over . Here I want to talk about some exponentiation-specific properties that aren’t part of the general properties. For example, the inverse of is and the inverse of is . The number 1 is its own inverse and the number 0 is the only number that doesn’t have an inverse because is not defined.

The distributive property can be used to rewrite expressions for a variety of purposes. When you are multiplying a number by a sum, you can add and then multiply. You can also multiply each addend first and then add the products together.
These are properties that, in a way, relate operations to each other. I’m going to address all these constraints in more detail in the next posts of this series. For now, let’s get back to the main properties. The first thing to note about addition is that this operation is valid for any two real numbers. As you’ll soon see, this isn’t true for all operations.
The Associative Properties of Addition and Multiplication
Arithmetic progression means a sequence of numbers in which each differs from the preceding one by a constant quantity. This difference is known as the common difference of that particular arithmetic progression. The common difference of an arithmetic progression is denoted by d. In Mathematics, a commutative property is the terms that aren’t needed while operating. This property is valid only for multiplication and addition processes. The properties of addition can be utilised to decrease the complex expressions in numerous algebraic equations.
Braskem S A : 2022 Annual Audited Financial Statements – Marketscreener.com
Braskem S A : 2022 Annual Audited Financial Statements.
Posted: Thu, 23 Mar 2023 06:13:07 GMT [source]
Thus the common difference is negative if b is a positive number and positive if b is a negative number. So if b is a positive number, the arithmetic progression is a decreasing one and if b is a negative number then it is an increasing one. Arithmetic progression is a sequence of numbers where the differences between two consecutive terms of a sequence remain the same.
What are the Properties of Operations in Arithmetic?
Meaning, logarithms like and are undefined. Now, technically, you could say something like but you’ll rarely see such expressions. The reason is related to the fact that mathematicians want to treat the logarithm as a continuous function. I’m going to tell you more about this in my upcoming posts about functions. Even though binary operations work with only two numbers, you can still have expressions containing more than one operation and more than two numbers. In such cases, the order in which you apply the separate operations may be important for the final result.
Like with roots, the validity of logarithms for numbers is directly related to exponentiation. First, the base cannot be 0 or 1 because those two numbers raised to any power return themselves. Which means expressions like and are undefined. So, when you raise a number to the additive inverse of another number , this results in the multiplicative inverse of .
You can see these constraints in the sections specific to each operation but we’re going to revisit this topic in the next posts of the series. You see that this also looks like a right distributive property but the division operation on the left becomes subtraction on the right. Distributive property, except the multiplication on the left became addition on the right. This also looks very much like a left distributive property, except the subtraction in the left-hand side became division in the right-hand side. The case for negative numbers is interesting. Normally, the convention is that logarithms don’t work with negative numbers (both as the base or as the number whose logarithm we’re taking).
There are four arithmetic operations such as addition, subtraction, multiplication, and division. Check your addition and subtraction, and think about the order in which you are adding these numbers. Use the commutative property to rearrange the addends so that compatible numbers are next to each other. Rewrite \(\ 7+2+8.5-3.5\) in two different ways using the associative property of addition. Show that the expressions yield the same answer. In other words, the placement of addends can be changed and the results will be equal.
Likewise, the commutative property of multiplication means the places of factors can be changed without affecting the result. The division is an inverse operation to multiplication. It has a quotient of two numbers i.e., dividend divided by a divisor. If the dividend is larger than the divisor, the quotient will be greater than 1 for positive numbers.

Well, it’s kind of like multiplication in reverse, so it is often considered to be the inverse of multiplication. Instead of adding multiple groups, you’re basically seeing what happens when you break a number into smaller groups. Let’s say you want to try subtraction instead, taking items away from a group.
Arithmetic Examples
Rewrite \(\ 52 \cdot y\) in a different way, using the commutative property of multiplication. Note that \(\ y\) represents a real number. However, recall that \(\ 4-7\) can be rewritten as \(\ 4+(-7)\), since subtracting a number is the same as adding its opposite. Applying the commutative property for addition here, you can say that \(\ 4+(-7)\) is the same as \(\ (-7)+4\). Notice how this expression is very different than \(\ 7-4\). Likewise, the commutative property of addition states that when two numbers are being added, their order can be changed without affecting the sum.
You will find that the associative and commutative properties are helpful tools in algebra, especially when you evaluate expressions. Using the commutative and associative properties, you can reorder terms in an expression so that compatible numbers are next to each other and grouped together. Compatible numbers are numbers that are easy for you to compute, such as \(\ 5+5\), or \(\ 3 \cdot 10\), or \(\ 12-2\), or \(\ 100 \div 20\). (The main criteria for compatible numbers is that they “work well” together.) The two examples below show how this is done. Multiplication has an associative property that works exactly the same as the one for addition.
- Properties of additional help in finding integers or result of adding them.
- We also have an app from which you can have a lot of amazing benefits.
- Remember, if parenthesis is involved, complete what is within the parenthesis first.
That is, if you have an expression with three numbers, the order in which you apply the operation doesn’t affect the final result. Which essentially allows you to drop the parentheses in the expression. Now that we know about all the important properties of an arithmetic progression, we will be able to solve all the sums related to an AP. We also have an app from which you can have a lot of amazing benefits. Get the testbook app today and start learning even on your phone.
For example, \(\ 30+25\) has the same sum as \(\ 25+30\). The associative, commutative, and distributive properties of algebra are the properties most often used to simplify algebraic expressions. You will want to have a good understanding of these properties to make the problems in algebra easier to solve. There are certain rules, or properties, that go along with addition.


